Here is Ilya's $0.821$-ellipse (if I interpreted his intention correctly),
discretized to an $180$-point polygon at $2^\circ$-degree angular
increments:
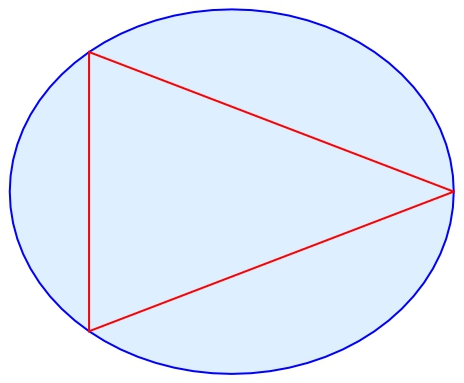
His point, if I may editorialize, is that the naive lower bound of
$4/\sqrt{\pi} \approx 2.26$ is not so far from the bound for the
optimal ellipse. My computations for this discrete version yield $\approx 2.97$,
in fact, slightly larger than $r=3 \sqrt{3/\pi}=2.93$$r=3 \sqrt{3/\pi} \approx 2.93$ for the circle.
Perhaps I miscalculated...
