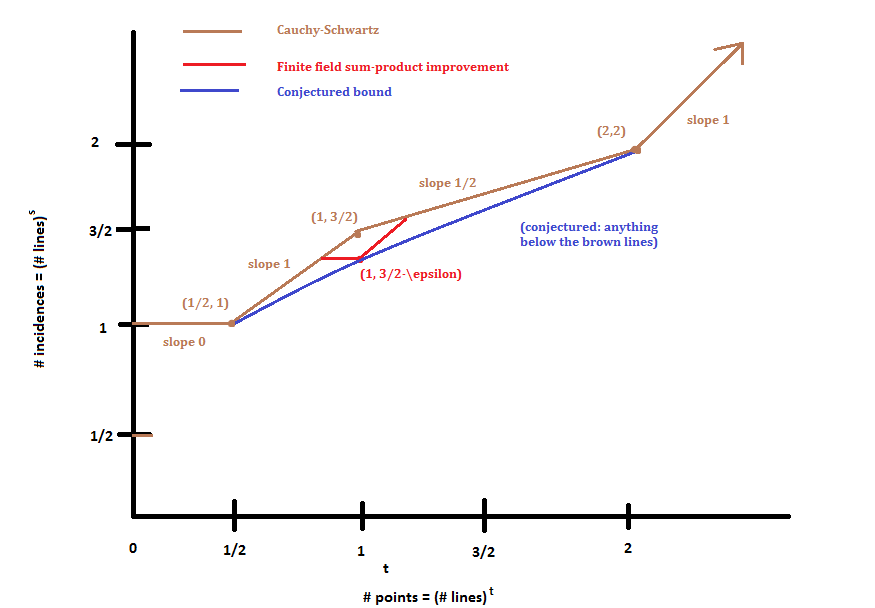
My question concerns the Szemerédi-Trotter theorem in $\mathbb{F}_q^2$. If we have $m$ points and $n$ lines in $\mathbb{F}_q^2$, then by Cauchy-SchwartzSchwarz the number of point-line incidences is as most $$\min(mn^{1/2}+n,\ m^{1/2}n+m). \qquad\qquad (1)$$ This is the brown line in the figure below.
We also know that if $m=n$, and $q^{\delta}<m<q^{2-\delta},$ then the number of incidences is at most $$ m^{3/2-\epsilon}\qquad\qquad\qquad (2) $$ for some $\epsilon=\epsilon(\delta)>0$. This is the red dot in the figure below.
Equation $(2)$ allows us to beat the bound $(1)$ provided $m$ is very close to $n$---just increase either $m$ or $n$ slightly so that $m=n$, and then apply $(2)$. This is the red line in the figure below.
My question is: are there any results that allow us to do better than the envelope of the brown and red lines---i.e. can we obtain any sort of bound that looks like the blue line below? My hope is that we can somehow interpolate between the red dot (in the figure below) and the two endpoint cases (the brown dots at $(1/2, 1/2)$ and at $(2,2)$.
Does anyone know of a result of this form, or does anyone have ideas on how to obtain such a result?
[edit: updated the picture to have correct numerology, and corrected (1)]
