Consider Toeplitz matrices where the entries in the first row and column (which define the whole matrix) are independently chosen to be either $1$ or $0$ with probability $1/2$. Define $p_n$ to be the probability that such a uniformly chosen $n$ by $n$ Toeplitz matrix is singular (over $\mathbb{R}$). Is it known that
$$\lim_{n\to \infty} p_n = 0 ?$$
The equivalent question for random Bernoulli matrices was resolved by Komlós (1963).
I see Probability of random (0,1) Toeplitz matrix being invertibleProbability of random (0,1) Toeplitz matrix being invertible where the exact value of $p_n$ was asked for (and with no answer to the part related to my question).
For $n = 1,\dots,11$ the number of singular matrices is $1,4,13,50,153,522,1648,5173,15047,43892, 123417$. Sadly this is not in OEIS. The following picture shows the probability that a random $0-1$ Toeplitz matrix is singular for increasing values of $n$.
Feb 6 2014: Fixed some of the numbers of singular matrices where the determinant was very close to zero.
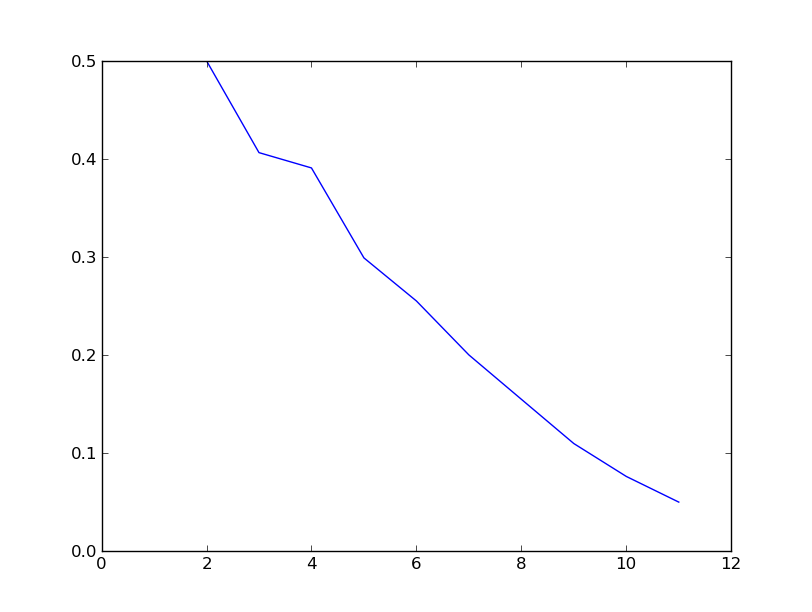
At the risk of asking a really trivial question, is it even obvious how to show that the probability is non-increasing?
