I am stuck in trying to interpret a definition in the paper "Categories of continuous functors" by P. Freyd and M. Kelly (click).
They say:
A category $\cal A$ with a proper factorization system $(\mathfrak E, \mathfrak M)$ [i.e. a factorization system where the left class is contained in the class $Epi$ and the right class in the class $Mono$ of monic arrows] has a generator when it has a small full subcategory $\cal G$ such that the family of all morphisms $G\to A$ with domain $G\in\cal G$ is in $\mathfrak E$. If $\cal A$ admits coproducts, then $\cal G$ is a generator iff the canonical arrow $\coprod_{G\to A}G\to A$ lies in $\mathfrak E$ for any $A\in\cal A$.
Edit: Proving that the two conditions are equivalent in presence of coproducts isseemed to be easy: one simply uses but trying to reproduce the universalargument I noticed that the diagram I used wasn't commutative. I wanted to say that the diagram
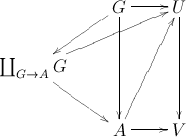
gives by lifting property of the coproductdesired arrow to show that each $G\to A$ lies in $\mathfrak E = {}^\perp\mathfrak M$.
What is seems incredible to me is that this notion is the right one to capture the notion of generator, or that of separator, in $\cal A$.
In fact, one of the main point of Freyd-Kelly's paper is that the two notions are not equivalent (as they are stated on the nlab or wikipedia, if I remember well): in a finitely complete -or discrete-cocomplete- category a generator separates arrows; with a particular choice of the factorization system, a separator is a generator.
My problem is that if I interpret "the family of all morphisms $G\to A$ with domain $G\in\cal G$ is in $\mathfrak E$" in the unique possible sense, I can't obtain what I expected: "each arrow $*\to X$ is an epi in $\bf Set$" is a blatantly false statement, even if in that case the terminal object separates arrows.
Can you help me? I feel I'm lost in something easy, but I don't see where.
