The computer found counterexamples to $\rho(G)<1$.
Despite verification, I am not sure this is correct.
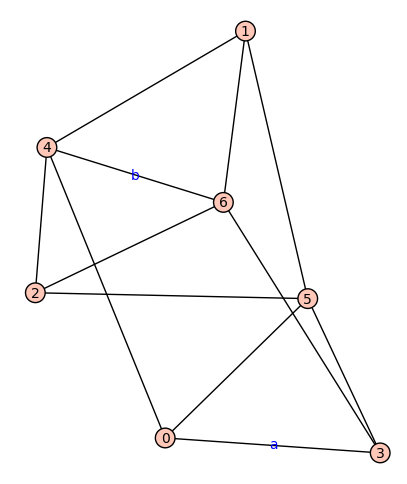
$G_1$ on $7$ vertices, $G_2$ on $11$ vertices. $\rho(G_1)=1,\rho(G_2)=2$
G_1$G_1$:
edges=[(0, 3), (0, 4), (0, 5), (1, 4), (1, 5), (1, 6), (2, 4), (2, 5), (2, 6), (3, 5), (3, 6), (4, 6)]
A=[(0, 3)]
B=[(4, 6)]
G_2$G_2$:
edges=[(0, 5), (0, 6), (0, 7), (1, 6), (1, 7), (1, 8), (2, 6), (2, 8), (2, 9), (3, 7), (3, 9), (3, 10), (4, 8), (4, 9), (4, 10), (5, 9), (5, 10), (6, 8), (7, 10)]
A=[(0, 5)]
B=[(6, 8), (7, 10)]
Plot of $G_1$: