Suppose one takes a large hexagonal region in the tiling of the plane by unit hexagons, with $n+1$ hexagons on each side, as seen in the figure below (taken from the COMAP website) for the case $n=5$.
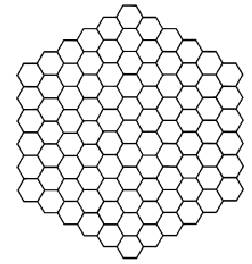
Starting from any corner cell (call it $C$) and proceeding cyclically, color $n$ consecutive boundary hexagons blue, the next $n$ yellow, the next $n$ blue, the next $n$ yellow, the next $n$ blue, and the next $n$ yellow. Now color the interior hexagons blue and yellow uniformly at random. This determines three (non-intersecting) percolation paths from the boundary of the region to itself, with yellow hexagons on one side of each path and blue hexagons on the other.
What is the probability, in the limit as $n \rightarrow \infty$, that the percolation path that starts next to $C$ will terminate at the diametrically opposite point on the boundary of the region?
I know that Smirnov et al. have proved theorems asserting conformal invariance for percolation on this lattice, but I don't know the technical details, so I don't know if all the hypotheses that those theorems require are satisfied here. Assuming the answer is "yes", then the question I'm asking is a special case of a much more general (and natural) question about a probability model associated with pairings of $2n$ points on the boundary of a disk, and I'd like to learn more about that question as well (though for my purposes, it really is the case $n=3$ with 6 equally-spaced points that I want to know about, and a closed-form expression or close approximation to the probability in question).
[Added after j.c.'s proposed solution: Note that the probability is less than 1/3, since (labeling the corners 1,2,3,4,5,6 in cyclic order) the events 1-is-connected-to-4, 2-is-connected-to-5, and 3-is-connected-to-6 are disjoint, have equal probability (by symmetry), and have total probability less than 1. So .4295722 is not a possible answer.]
