Just a bit more "data" in the form of images. I started with a "random polyhedron"
built from the convex hull of a small number of points. Then I iterated the process
of replacing each face by its centroid, i.e., $\alpha=\beta=\gamma=\frac{1}{3}$
in my original description, and
again taking the convex hull. At least visually, the data support Gjergji's suggestion
that the process converges to ellipsoids (of different axes lengths).
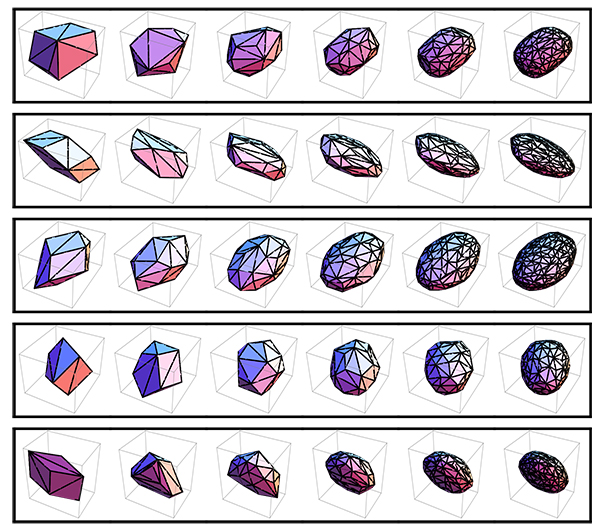
So, to make an explicit conjecture out of these observations:
Conjecture. Given any convex polyhedron $P$ in $\mathbb{R}^3$, let $c(P)$ be the convex hull of the centroids of the faces of $P$. Then $c^k(P)$ converges to an ellipsoid as $k \to \infty$.
Secondarily, it makes sense to conjecture the same holds for any convex polytope $P$ in $\mathbb{R}^d$.
