Consider the function $f : \mathbb{R} \to [0,1]$$f : \mathbb{R} \to [-1,1]$ with
$$
f(x) = \begin{cases}
-1 & x \le -1 \\
+1 & x \ge +1 \\
\frac{f(\frac32 (x-\frac13)) + f(\frac32 (x+\frac13))}{2} & -1 \le x \le +1\,.
\end{cases}
$$
So $f$ is the average of two affine transformations of itself.
The picture shows the graph of $f$ (in red) and the two affine transformations from the definition (in blue).
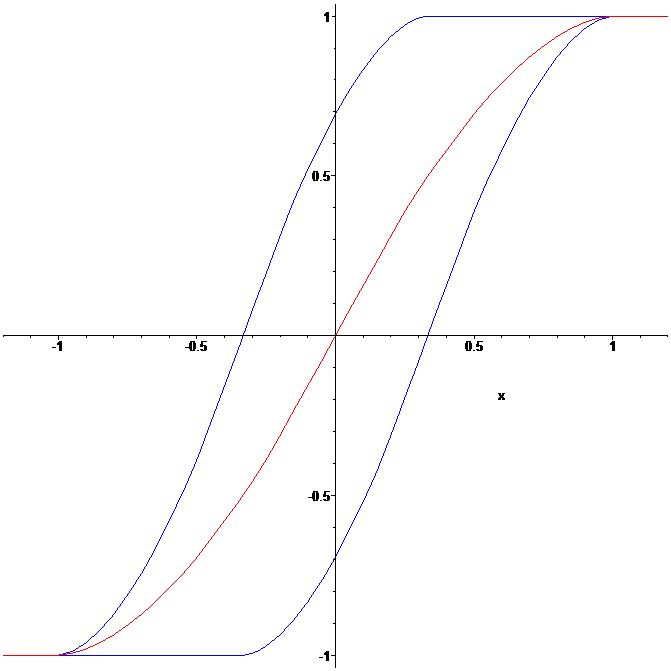 What is known about such functions? Is $f(0.5) \approx 0.694064$ rational?
What is known about such functions? Is $f(0.5) \approx 0.694064$ rational?
