Here is one piece of software, the Book Knot Simplifier, by Maria Andreeva, Ivan Dynnikov, Sergey Koval, Konrad Polthier, and Iskander Taimanov, largely based on Dynnikov's work:
Here we offer a set of tools for manipulating knots and links in the three-space by using the three-page presentation of links, which was proposed and developed by I. Dynnikov in
[1][2][3].
The approach is based on a simple and very well known observation that every link in the three-space is topologically equivalent to a link that lies entirely in a three-page book, i.e. the union of three half-planes with common boundary. Though being not convenient for human perception, this way of presenting links seems to be very efficient for handling knots by computer. It provides a very quick way from a combinatorial description of a link to its three-dimensional presentation. A three-page link admits a lot of transformations that preserve its isotopy class and can be easily found. This fact is used in the knot simplifying tool included herein.
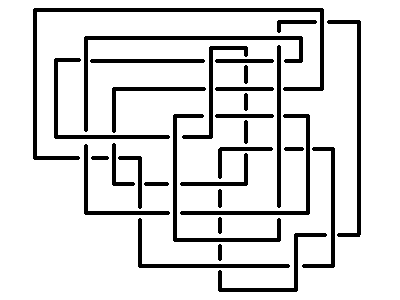
The unknot with 31 crossings.
