For $k=5$, is this the optimal partition?
Rectangle sides
$x=\frac{1}{6} \left(3-\sqrt{3}\right) \approx 0.21$ and $1-x$,
and (now corrected) all diagonals of length$^2$ of $\frac{2}{3}$, and so length $\sqrt{2/3} \approx 0.816$.
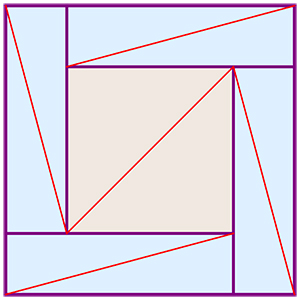
And here is Wlodzimierz's much better partition. Each diagonal has length $\sqrt{2257}/72 \approx 0.660$:
![WK5Rects][2]
For $k=8$, the $4 \times 2$ partition has diagonal $\sqrt{5}/4 \approx 0.559$. Here are two more partitions. Left is rational; right isa better but is, irrational partition, $x=\frac{2}{3}-\frac{\sqrt{\frac{7}{3}}}{6} \approx 0.412$:
![Sq8Rects][3][Sq8Rects62][3]
