This is more of a general comment on the direction of multiple category theory rather than an answer to the question but I hope it will be useful in directing attention to the origins of some of these questions in homotopy theory and for suggesting lines, or a line, for further development. In this I will possibly still continue Out of LineOut of Line!
I looked at the Berner-Rezk paper referred to in the above, and found the following diagram for compositions in $2$-categories.
comp http://pages.bangor.ac.uk/%7Emas010/2catcomp.jpg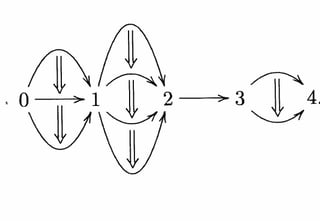 (source)
(source)
Compare this with the simplicity of describing the following in a double category.
dopuble http://pages.bangor.ac.uk/%7Emas010/array.jpg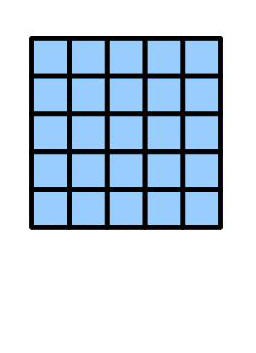 (source)
(source)
One writes this as an array $(a_{ij})$ with composition $[a_{ij}]$, and this notation is easily extended to $n$ dimensions without using operads, though these might be useful in the cubical context. Note also that an inverse in a specific direction is easy to envisage.
This is why I have found it very difficult to conjecture and prove results in the globular setting, while the cubical setting has allowed a new approach to basic algebraic topology using filtered spaces and homotopy classes of mappings.
My writing to Alexander Grothendieck in 1982 did stimulate his writing of "Pursuing Stacks" in 1983, but he noted that the model of $\infty$-groupoids did not model all homotopy types, and proceeded to look for weak categorical models, which are clearly important. But when in 1985 (or 6) I told him of Loday's work that (strict) $n$-fold groupoids model homotopy $n$-types, he exclaimed: "That is absolutely beautiful!" This work relates to classical parts of homotopy theory, such as $n$-ads, and the Blakers-Massey and Barratt-Whitehead theorems, and gives some new explicit calculations of homotopy $n$-types in a nonabelian context.
Another aspect of Loday's work is his notion of "resolution" of a space: the first stage resolves in one direction, the second in a different direction, and so on, ending up with a resolution of spaces by $n$-cubes of fibrations, which has been clearly explained by Richard Steiner, J. London Math. Soc. (2) {34} (1986) 169--176.
What I am arguing for is an eclectic approach, in which the advantages and difficulties of various approaches are considered.
Update: I'll add a reference to J.C. Morton, "cubical $n$-categories and finite-limits theories", arxiv: 1001.2628, and some further references there. Another relevant paper is arXiv:1204.5101 [pdf, ps, other] n-Fold groupoids, n-types and n-track categories, David Blanc, Simona Paoli
September 1, 2014 Here is a link to a presentation I gave at the IHP, Paris, June 5, 2014 on Intuitions for cubical methods in nonabelian algebraic topology Intuitions for cubical methods in nonabelian algebraic topology) (Wayback Machine).
December 26, 2015 I would also like to add this presentation in June 2015 at Aveiro on
A philosophy of modelling and computing homotopy typesA philosophy of modelling and computing homotopy types (Wayback Machine),
which illustrates the aim of doing specific nonabelian colimit computations in homotopy thoerytheory.
October 26, 2016 A writeup of part of the above presentation is available on the arXiv as Modelling and Computing Homotopy Types:I.
