Have a look at
(R. BROWNBrown, PP.R. HEATHHeath and H. KAMPSKamps), ``Groupoids"Groupoids and the Mayer-Vietoris sequence''sequence", J. Pure Appl. Alg. 30 (1983) 109-129.
A Mayer-Vietoris sequence for a pullback of a covering mao also appears in Section 10.7 of Topology and GroupoidsTopology and Groupoids, and was in the 1988 (differently named) edition.
Edit: Here is an extract from the above paper
mv http://pages.bangor.ac.uk/%7Emas010/gpds-mv.jpg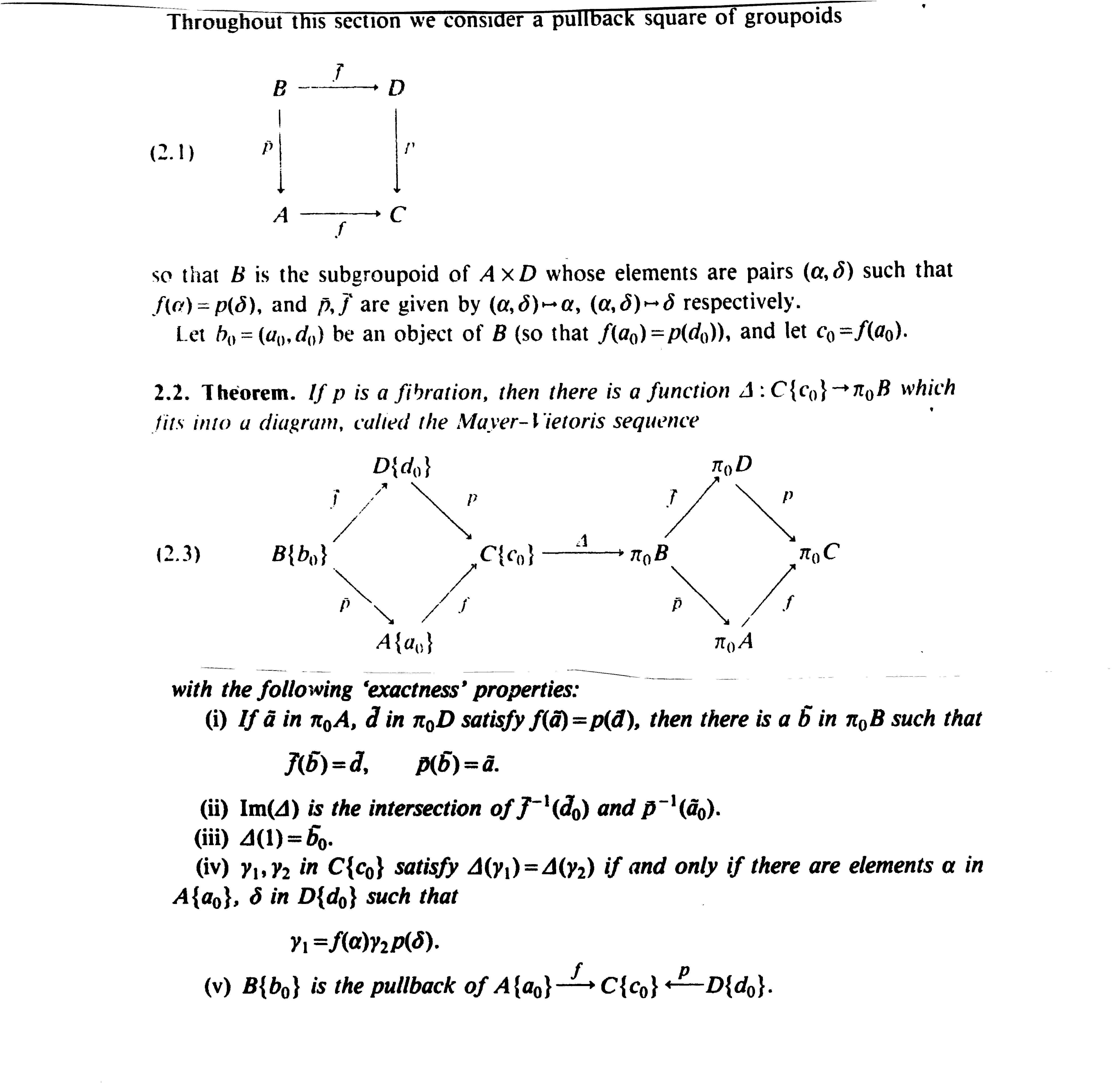 (source)
(source)
which shows that there is some more information from the sequence than just the usual exact sequence. This sequence applies to spaces as is shown in Section 4 of the above paper. The point is that this detailed exactness is easier to extract in the groupoid model than directly in the topology.
