By a drawing of the Fano plane I mean a system of seven simple curves and seven points in the real plane such that
- every point lies on exactly three curves, and every curve contains exactly three points;
- there is a unique curve through every pair of points, and every two curves intersect in exactly one point;
- the curves do not intersect except in the seven points under consideration.
The familiar picture
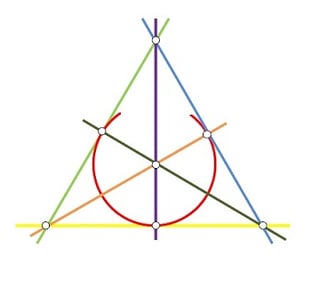 Traditional Fano plane http://math.haifa.ac.il/%7Eseva/MathOverflow/Fano-1.jpg(source)
Traditional Fano plane http://math.haifa.ac.il/%7Eseva/MathOverflow/Fano-1.jpg(source)
does not count as a drawing, since the last requirement is not satisfied: there are two "illegal" intersections. In fact, this is easy to fix:
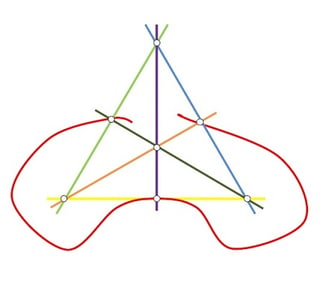 Non-intersecting Fano plane http://math.haifa.ac.il/%7Eseva/MathOverflow/Fano-2.jpg(source)
Non-intersecting Fano plane http://math.haifa.ac.il/%7Eseva/MathOverflow/Fano-2.jpg(source)
However, this drawing is degenerate in the sense that two of the curves just "touch" each other, without crossing, at some point. And here, eventually, my question goes:
Is every drawing of the Fano plane degenerate?
(Although I can give a topological definition of degeneracy, it is a little technical and, may be, not the smartest possible one, so I prefer to suppress it here.)
