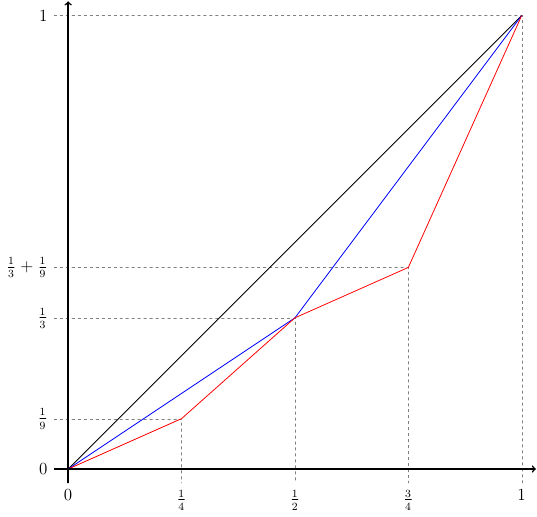
The infimum can be zero as pointed out by Anton Petrunin. Here's a construction on the interval $[0,1]$.
Consider a sequence of piecewise linear functions $f_n:[0,1] \to [0,1]$ each of which is strictly increasing defined by (see figure):
- $f_0(x) = x$ for all $x$.
- $f_{n+1}$ coincides with $f_n$ on diadic numbers of denominator $2^{-n}$.
- $f_{n+1}\left(\frac{2k+1}{2^{n+1}}\right) = f_n\left(\frac{k}{2^n}\right) + \frac{1}{3^{n+1}}$ for integer $k$.
Let $f = \lim\limits_{n \to +\infty}f_n$ and $d_1$ be the pullback of the standard Euclidean distance on $[0,1]$ under $f$. One has that
$$d_1(0,1/2) = \frac{1}{3}$$ $$d_1(0,1/4) = d_1(1/2,3/4) = 1/9$$ $$d_1(0,1/8) = d_1(1/4,3/8) = d_1(1/2,5/8) = d_1(3/4,7/8) = 1/27$$ etc...
One can construct in similar fasion a distance $d_2$ satisfying $$d_2(1/2,1) = \frac{1}{3}$$ $$d_2(1/4,1/2) = d_2(3/4,1) = 1/9$$ $$d_2(1/8,1/4) = d_2(3/8,1/2) = d_2(5/8,3/4) = d_2(7/8,1) = 1/27$$ etc...
By considering diadic partitions one sees that the infimum is $0$ when one can use the distances $d_1$ and $d_2$.