Some empirical data, with each point the average of 50 trials:
MinDistSquare http://cs.smith.edu/~orourke/MathOverflow/MinDistSquare.jpg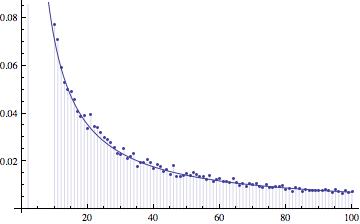
The curve $1/(\sqrt{2} n)$ (displayed) fits rather well. Congrats to Douglas Zare and Ori Gurel-Gurevich!
