This is the (finite) covering problem in the plane. According to Boroczky'sBöröczky's book "Finite packing and covering"Finite packing and covering, the answer is only known (provably) up to $k = 10$, due to the work of K. Bezdek and G. Fejes Toth. I don't know if the solutions are a famous sequence of geometric graphs.
http://mathworld.wolfram.com/images/eps-gif/DiskCoveringProblem5_800.gif
Copied image to imgur.com, as it was not being displayed because of the new https rule. Added link to original image source.
jeq
- 1.2k
- 5
- 16
- 21

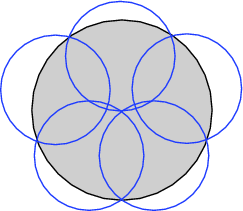 (Image from
(Image from