Return to Answer

First, a simple position with value $\omega$. The main line of play here calls for black to move his center rook up to arbitrary height, and then white slowly rolls the king into the rook for checkmate. For example, 1...Re10 2.Rf5+ Ke6 3.Qd5+ Ke7 4.Rf7+ Ke8 5.Qd7+ Ke9 6.Rf9#. By playing the rook higher on the first move, black can force this main line of play have any desired finite length. We have further variations with more black rooks and a white king.

First, a simple position with value $\omega$. The main line of play here calls for black to move his center rook up to arbitrary height, and then white slowly rolls the king into the rook for checkmate. For example, 1...Re10 2.Rf5+ Ke6 3.Qd5+ Ke7 4.Rf7+ Ke8 5.Qd7+ Ke9 6.Rf9#. By playing the rook higher on the first move, black can force this main line of play have any desired finite length. We have further variations with more black rooks and a white king.

This is a great question, which I have been pondering for some time.

alt text http://jdh.hamkins.org/files/2013/02/Value-omega-290x300.jpg

alt text http://jdh.hamkins.org/files/2013/02/Value-omega-squared-300x187.jpg

alt text http://jdh.hamkins.org/files/2013/02/Releasing-the-hoards-300x246.jpg

alt text http://jdh.hamkins.org/files/2013/02/Omega-squared-times-41-300x174.jpg

We can arrange the towers so that black may in effect choose how many rook towers come into play, and thus he can play to a position with value $\omega^2\cdot k$ for any desired $k$, making the position overall have value $\omega^3$.

alt text http://jdh.hamkins.org/files/2013/02/Value-omega-cubed-1024x744.jpg

alt text http://jdh.hamkins.org/files/2013/02/Climbing-the-stairs-1024x206.jpg

My co-author Cory Evans holds the chess title of U.S. National Master.

Update. (Oct 28, 2015) See below, for a position with game value $\omega^4$.

This is a great question, which I have been pondering for some time.

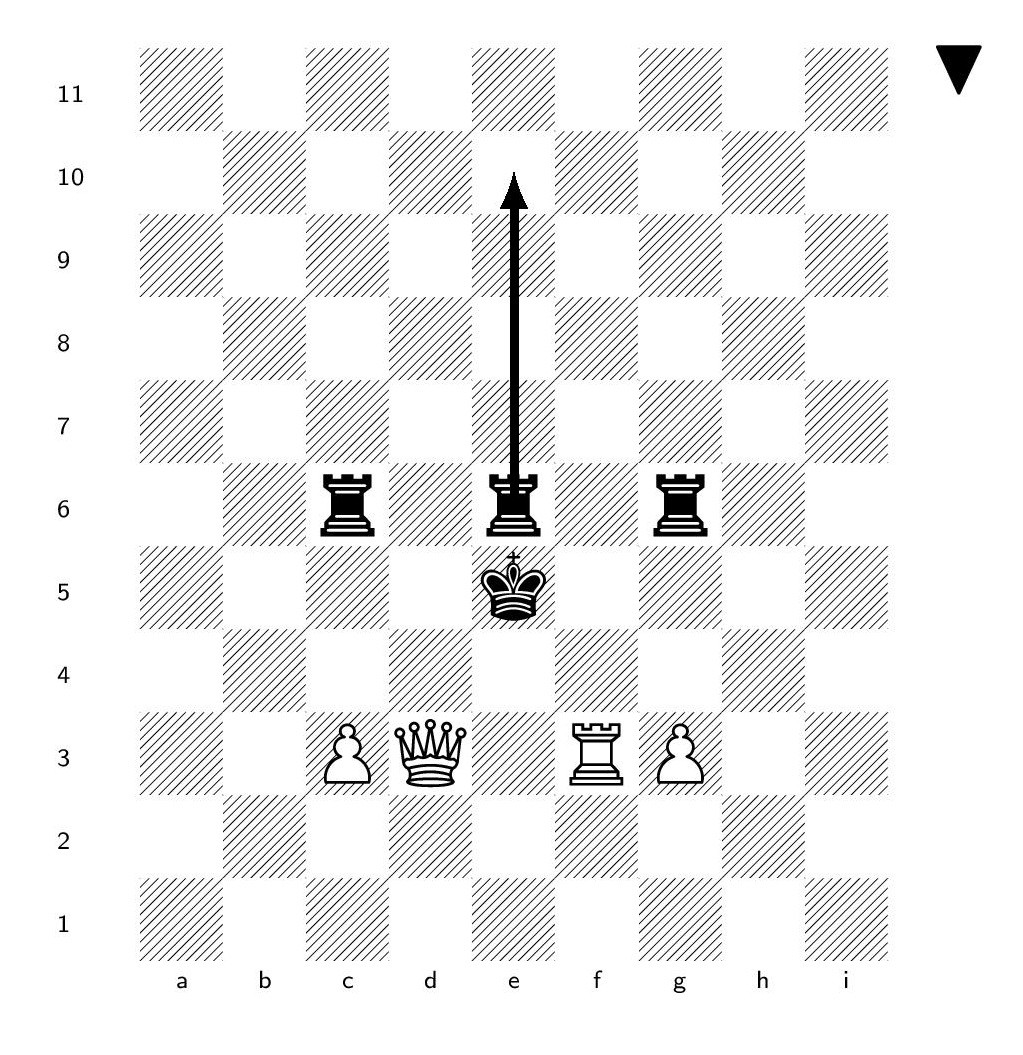
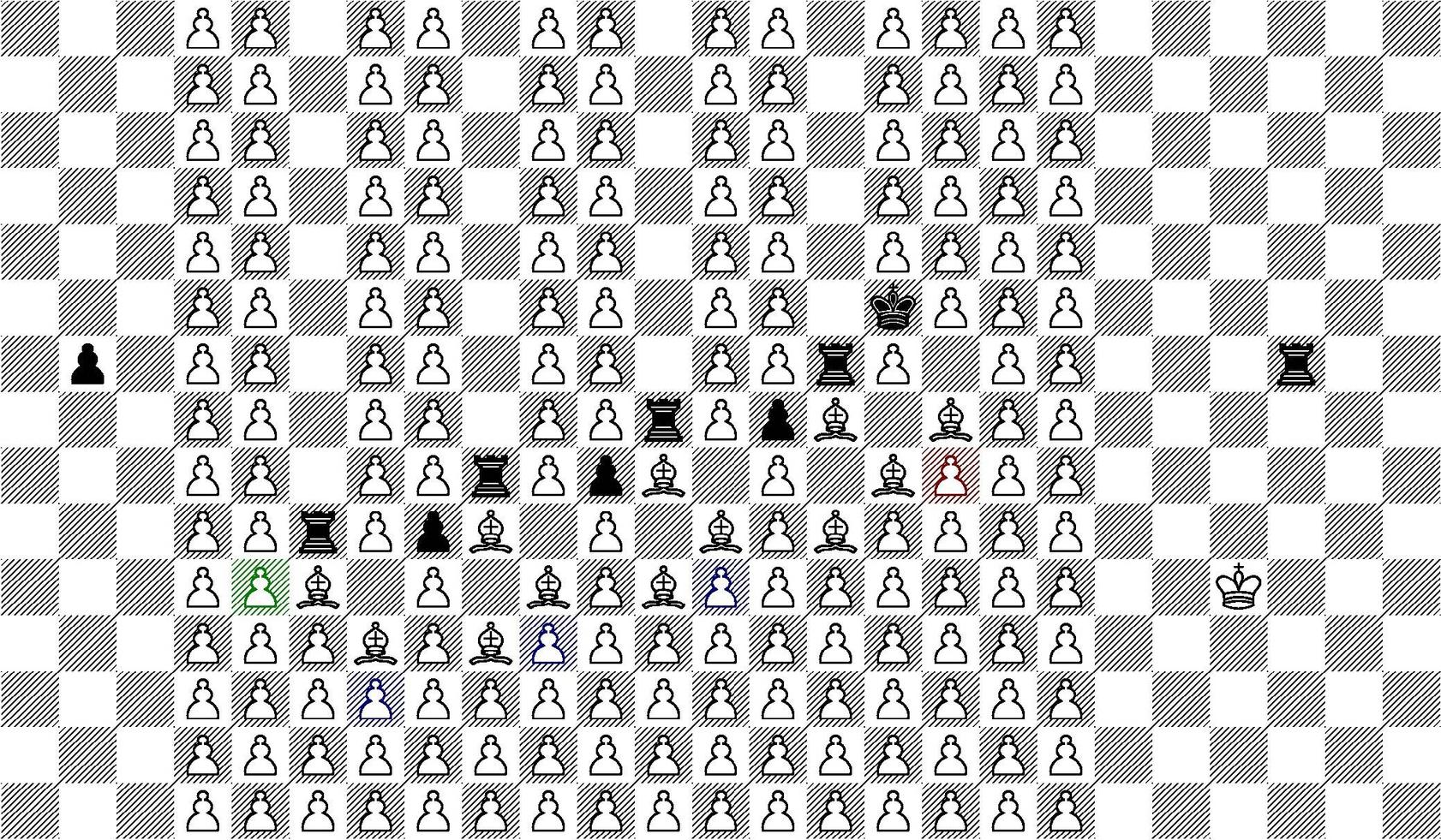
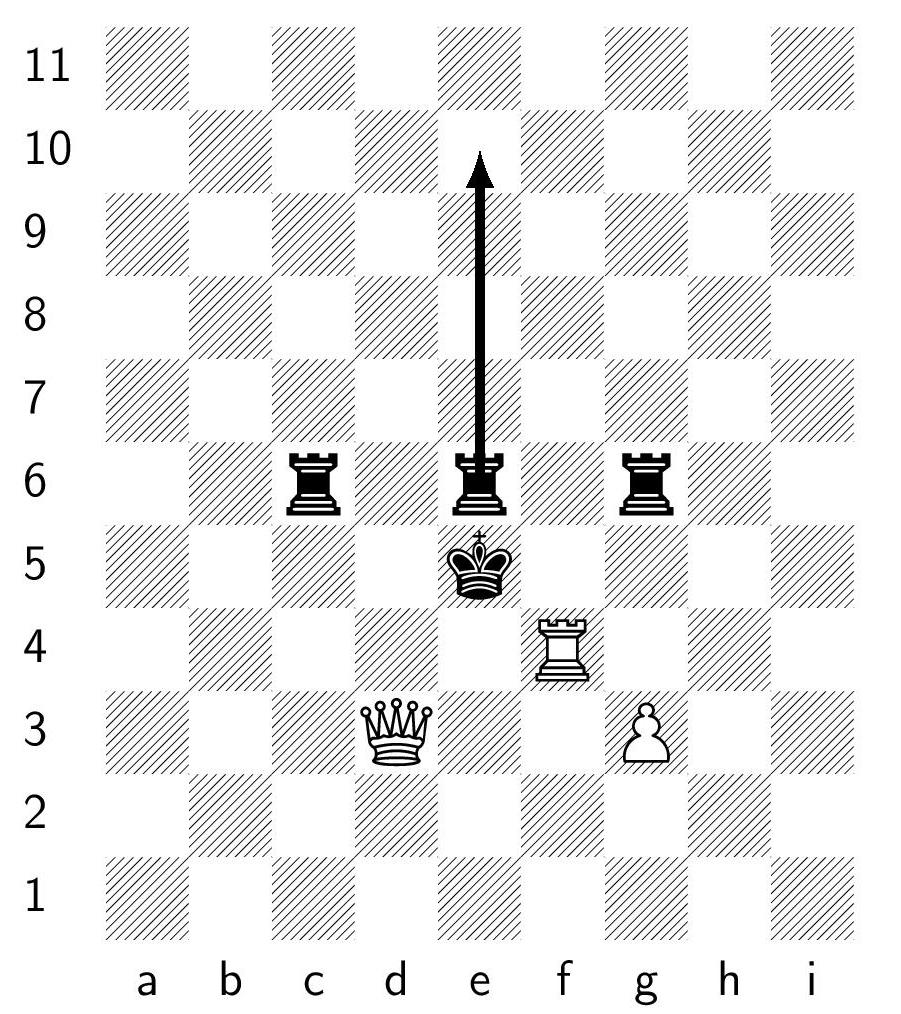
We can arrange the towers so that black may in effect choose how many rook towers come into play, and thus he can play to a position with value $\omega^2\cdot k$ for any desired $k$, making the position overall have value $\omega^3$.

My co-author Cory Evans holds the chess title of U.S. National Master.

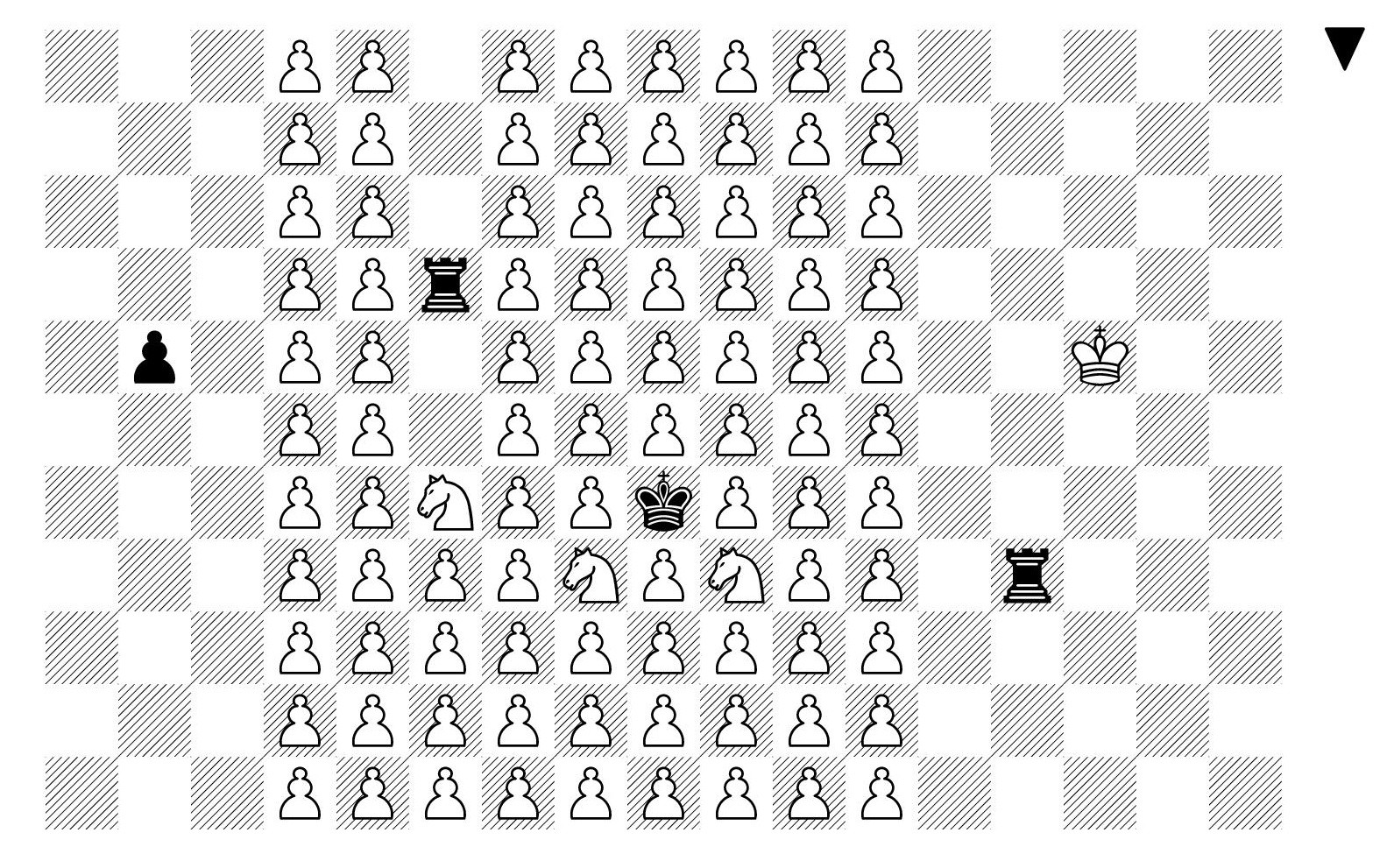
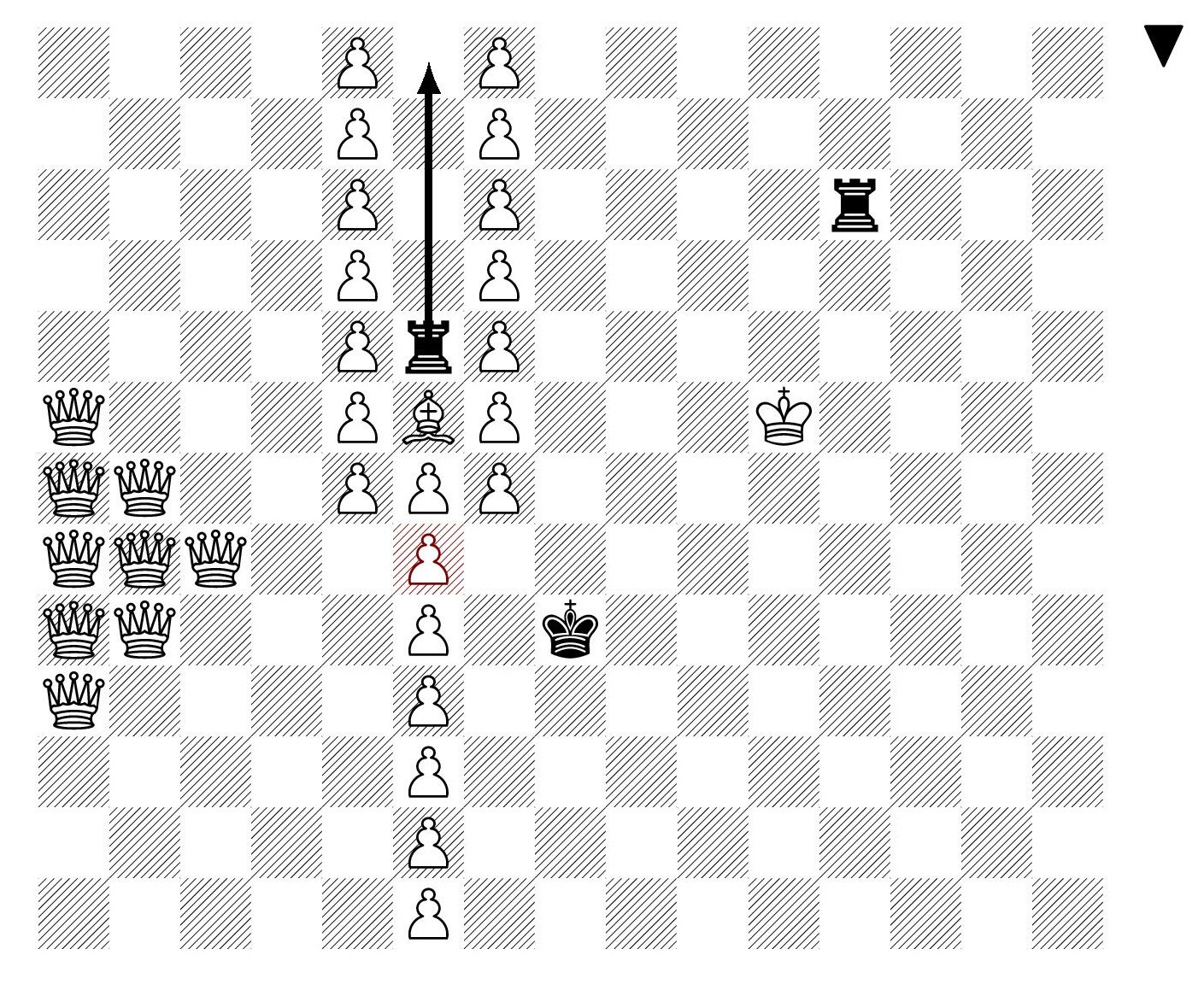
Update. In new joint work, we've found a position with game value $\omega^4$.

• C. D. A. Evans, J. D. Hamkins, and N. L. Perlmutter, A position in infinite chess with game value $\omega^4$.

In this position, the kings sit facing each other in the throne room, an uneasy détente, while white makes steady progress in the rook towers. Meanwhile, at every step black, doomed, mounts increasingly desperate bouts of long forced play using the bishop cannon battery, with bishops flying with force out of the cannons, and then each making a long series of forced-reply moves in the terminal gateways. Ultimately, white wins with value $\omega^4$.

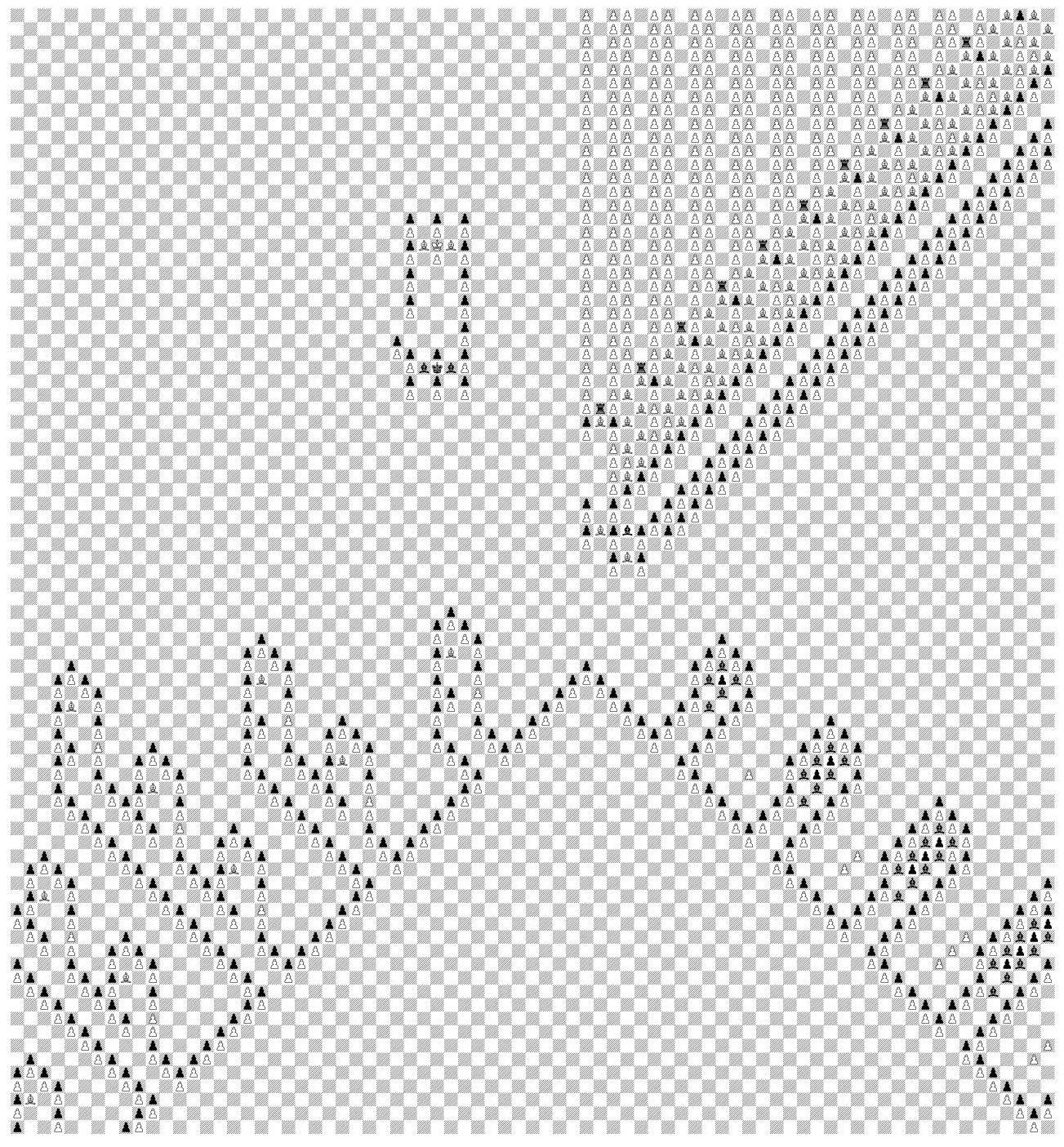
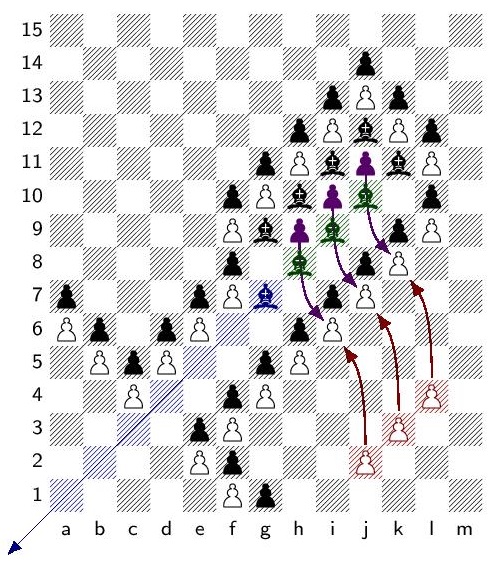
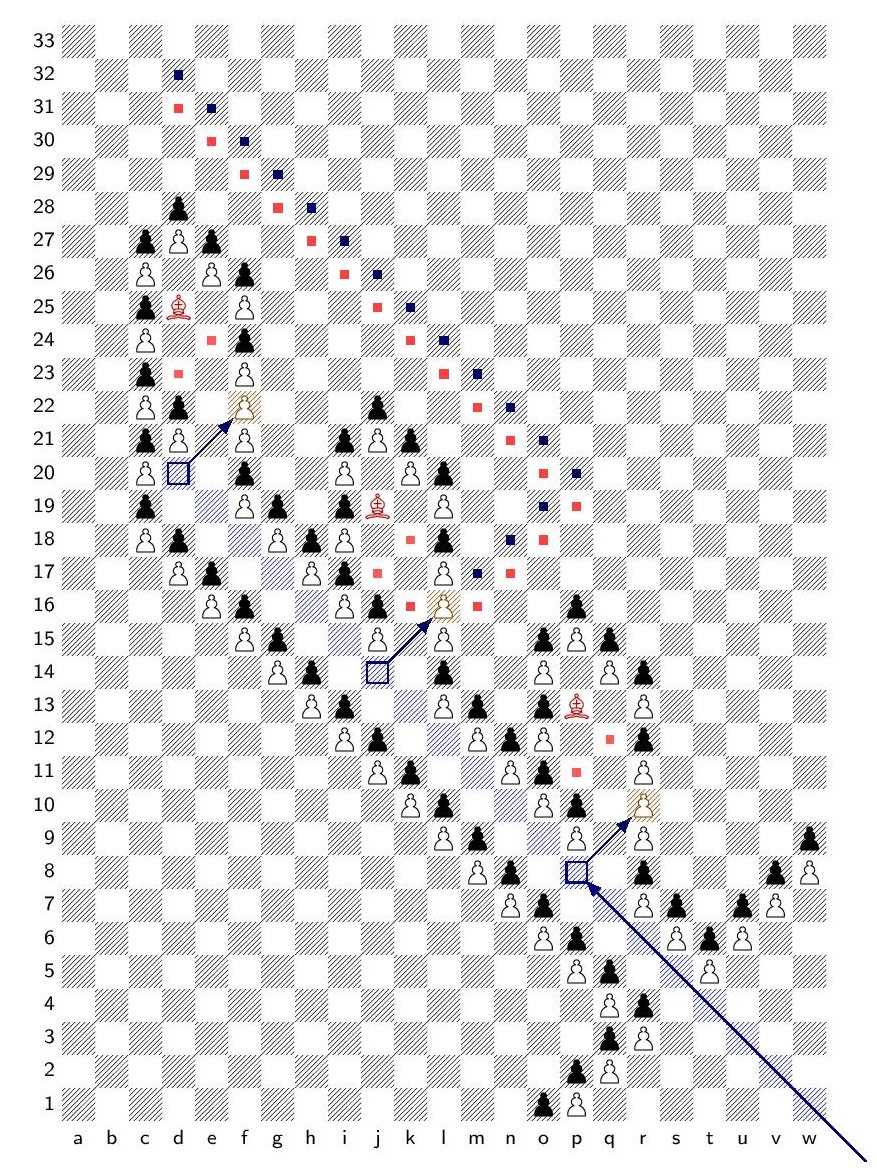
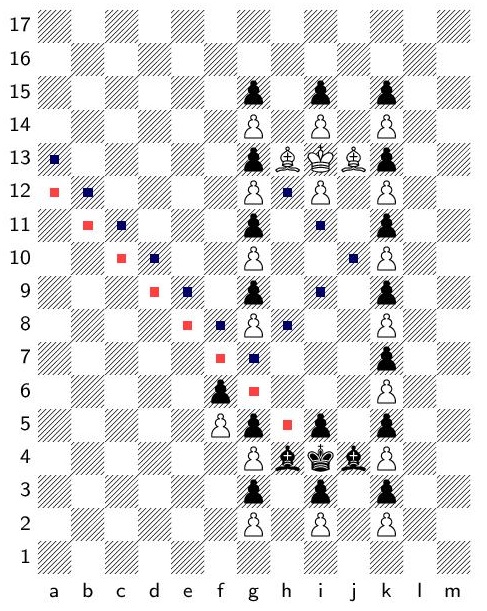
The position is fully explained in the article (click through to the arxiv for the pdf).

Here is a similar position with value $\omega^2$, which we call, "releasing the hoards", since white aims ultimately to open the portcullis and release the queens into the mating chamber at right. The black rook ascends to arbitrary height, and white aims to advance pawns, but black embarks on arbitrarily long harassing check campaigns to delay each white pawn advance.

Here is a similar position with value $\omega^2$, which we call, "releasing the hordes", since white aims ultimately to open the portcullis and release the queens into the mating chamber at right. The black rook ascends to arbitrary height, and white aims to advance pawns, but black embarks on arbitrarily long harassing check campaigns to delay each white pawn advance.