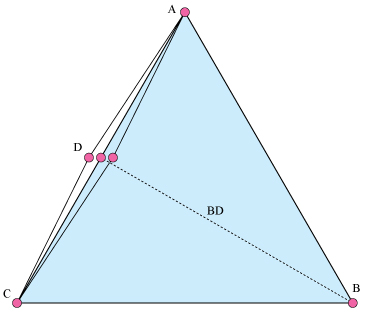
No. (This was an answer to a previous, not entirely clear version of the problem.) Take an equilateral triangle ABC of side length 1, plus the midpoint D of the side AC.
By pushing D slightly in or out parallel to the line BC, you can make ABCD nonconvex or convex. BD is the only irrational distance among the 6 distances. By taking the perturbation sufficiently small (say $\pm0.01$), you cannot distinguish from sums and differences whether BD is larger or smaller than in the original (degenerate) position.
alt text http://cs.smith.edu/%7Eorourke/MathOverflow/GunterQuad.jpg