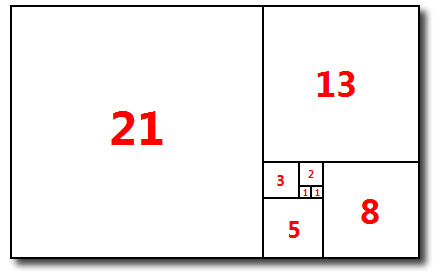
There are a couple of Fibonacci identities, I think. For example
$F_0^2+F_1^2+\cdots+F_n^2=F_{n}F_{n+1}$, with $F_0=1$.
By putingputting together squares of side $F_n$, one at a time, you get a rectangle of dimension $F_nF_{n+1}$: The two squares of side 1, then the square of side 2, then the square of side 3 and so on.
Here is an image I found online