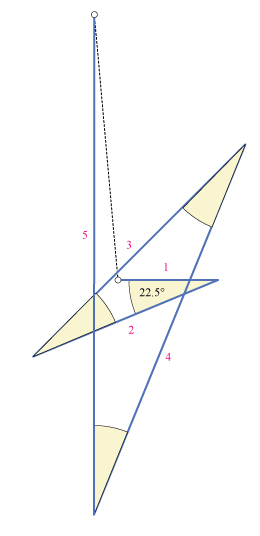
"Place $l_{2}$ at either of the end points of $l_{1}$, making an angle of $\alpha_{1}$"...
Making an angle $\alpha_{1}$ with respect to which line, measured in which direction? Here is a possible example with $\alpha_i=\pi/8$, but until you remove the ambiguity in your stipulation, it is unclear
if this accord with your intention:
Lengths and Angles http://cs.smith.edu/~orourke/MathOverflow/LengthsAndAngles.jpg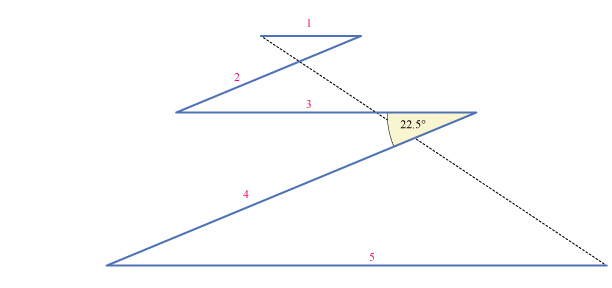
The OP has now made clear that the above fails his definition.
So, if $\alpha_i$ is a constant, the distance is entirely determined.
For $\alpha_i=\pi/8$, here is the determined shape and so end-to-end distance:
Lengths and Angles: 2nd version http://cs.smith.edu/~orourke/MathOverflow/LengthsAndAngles2.jpg