array http://pages.bangor.ac.uk/%7Emas010/array.jpg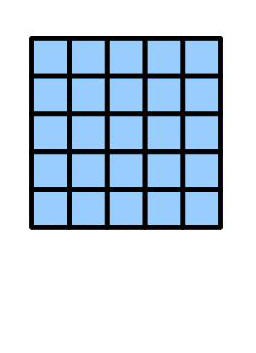 (source)
(source)
@David: An advantage of strict $n$-fold categories is that they can express in a simple way the above diagram in the form of "the big square is the composition of the little squares"; namely one defines a composable array $(a_{ij})$ to be an array of elements such that each is composable with its immediate neighbours. Then by associativity and the interchange law, the composite $[a_{ij}]$ is well defined. This "algebraic inverse to subdivision", which is easily extended to higher dimensions, is used extensively by Higgins and me in proving a Higher Homotopy Seifert-van Kampen theorem, in the paper available herehere. I do not know how to develop analogous methods in the globular or simplicial contexts; simplicial methods, but not of the higher categorical type, are used by Brown and Loday to prove their version of a HHSvKT. All these bring nonabelian colimit methods into homotopy theory.
I'll also mention that Brown and Higgins proved a groupoid result on the equivalence between strict cubical and globular $\omega$-groupoids, and the analogous but more difficult category result was proved by Al-Agl, Brown, and Steiner. The references are on the (updated) ncatlab page given by David.
Later: for complete clarity, though I expect it will be clear, I just add that if $a_{ij}$ is not at the edge of the square, then its immediate neighbours are: $a_{i-1,j}, a_{i+1,j}, a_{i,j-1}, a_{i,j+1}$, and the rule for composability of two squares is that their appropriate edges agree. My papers with Higgins contain lots of 2-dimensional rewriting, using the interchange law; and the paper with Al-Agl and Steiner contains a 3-dimensional rewriting argument to obtain a braid relation.
