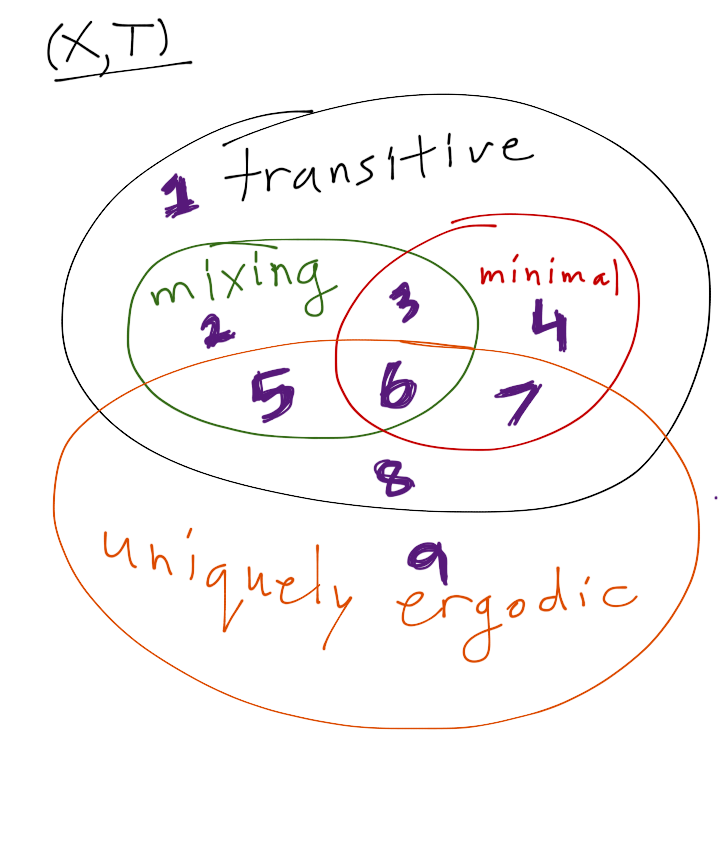
3. Constructed by Bassam Fayad, Topologically mixing and minimal but not ergodic, analytic transformation on $\mathbb{T}^5$Topologically mixing and minimal but not ergodic, analytic transformation on $\mathbb{T}^5$, 2000.
4. Constructed by Furstenberg, Strict ergodicity and transformation of the torusStrict ergodicity and transformation of the torus, 1961.
6. As Ian Morris points out in the comments, the identity map on a singleton set works here. A less trivial example was given by Karl Petersen, A topologically strongly mixing symbolic minimal setA topologically strongly mixing symbolic minimal set, 1970.