Not an answer, just illustrations from some quick simulations.
Immediately below, the previous position of the walker is blocked with probability
$\frac{1}{2}$, and blocked for just one time step:
Walk Barrier 100 steps http://cs.smith.edu/%7Eorourke/MathOverflow/WalkBarrier100_1.jpg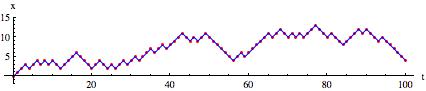
Below, again blocked with probability
$\frac{1}{2}$, but now blocked for two time steps:
Walk Barrier 100 steps http://cs.smith.edu/%7Eorourke/MathOverflow/WalkBarrier100_2.jpg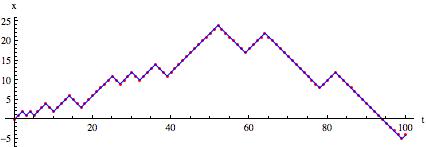
And now three time steps:
Walk Barrier 100 steps http://cs.smith.edu/%7Eorourke/MathOverflow/WalkBarrier100_3.jpg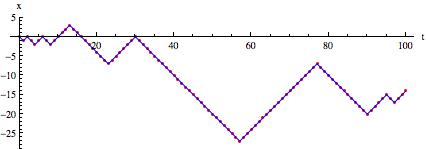
Of course, in this model, it is quite possible to get "stuck," if the barriers last long
enough (4 or more time steps in my simulation).
