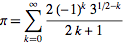According to constructivism, "it is necessary to find (or "construct") a mathematical object to prove that it exists". There are several formulas to calculate pi$\pi$, such as:
so I take it pi$\pi$ exists according to constructivism.
According to finitism, which is a form of constructivism, "a mathematical object does not exist unless it can be constructed from natural numbers in a finite number of steps."
Where does that leave irrational numbers, such as pi$\pi$? Do they simply not exist according to finitism? How does one reason about the ratio between a circle's circumference and its diameter, if one is working within a finitistic/ultrafinitistic framework?