There exists examples of different point configurations in $R^2$$\mathbb{R}^2$ having the same the set (but different matrices of!)
distances. The simplest example contains 4 points and could be found in the paper of Boutin and Kemper, see http://arxiv.org/pdf/math/0304192v1.pdf -- scroll to page 5 to see the picture
[Added by J.O'Rourke]:
Fig4 http://cs.smith.edu/%7Eorourke/MathOverflow/FourPointConfig.png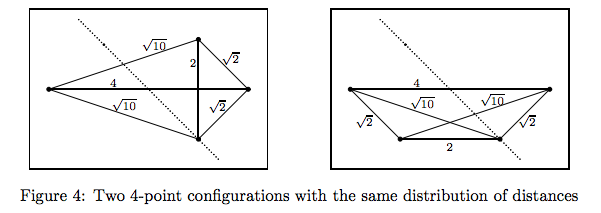
It is shown though (also Kemper, I believe) that for most configurations the set of distances determine the configuration (which is probably intuitively expected).
The example I have mentioned answers youyour question, but actually it would be natural if in your question you also require that the distances come with their multiplicities.
