In Higher Algebra by Lurie, we define the absolute cotangent complex $L_{A}$ through the composition $C\stackrel{\triangle}{\longrightarrow} Fun(\triangle^{1},C)\stackrel{F}{\longrightarrow}T_{C}$ where $T_{C}$ is the tangent bundle to $C$,and the functor $F$ restricts to the fiber over $B$ as the infinite suspension functor $\sum^{\infty}: C_{/B}\rightarrow Sp(C_{/B})$. And the absolute cotangent complex $L_{A}$ is exactly the infinite suspension of $B\stackrel{id}{\longrightarrow} B\in C_{/B}$ because the diagonal functor sends $B\in C$ to identity map in $Fun(\triangle^{1},C)$.
And so my $\textbf{question}$ is:
$\textbf{Is the relative cotangent complex $L_{B/A}$ the infinite suspension of $A\rightarrow B\in C_{/B}$?}$
Here is my attempt on this question:
The relative cotangent complex $L_{B/A}$ is identified with the absolute cotangent complex in $B\in C_{A/}$ by corollary 7.3.3.15 in HA, so we can see that the relative cotangent complex $L_{B/A}$ is the infinite suspension of $(A\rightarrow B)\stackrel{id}{\longrightarrow} (A\rightarrow B)\in (C_{A/})_{/B}$, so I need to show that such inifinite suspension coincides with the suspension of $A\rightarrow B\in C_{/B}$.
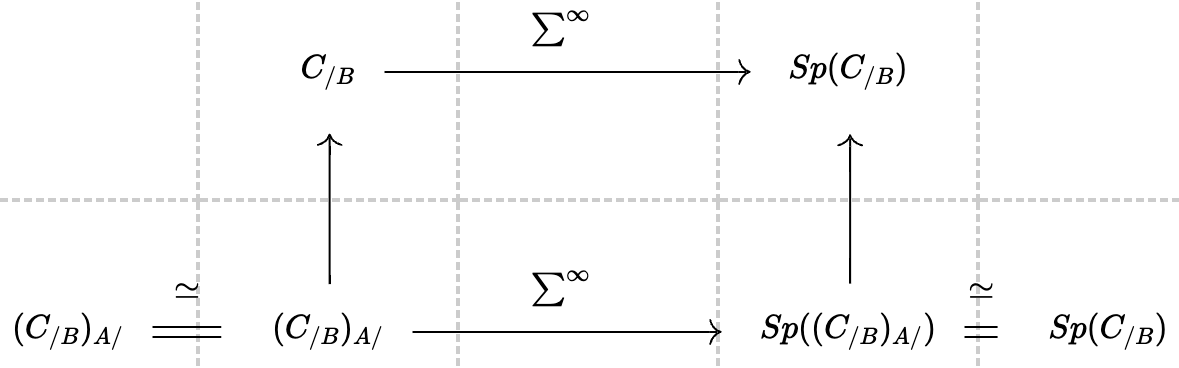
In short, it suffices to prove the existence of the following commutative diagram:
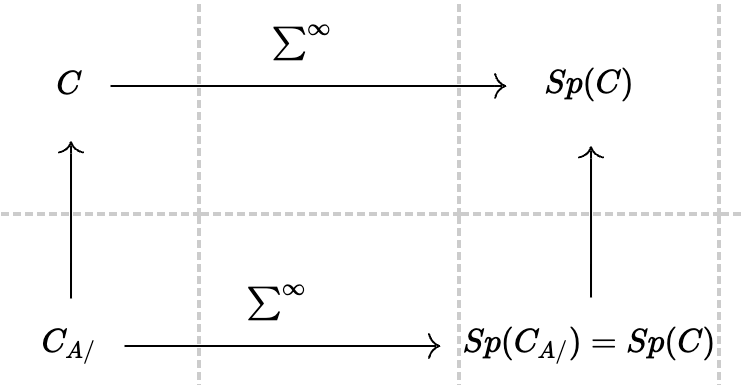
It also suffices to prove the existence of the following diagram:
,which seems to be some properties of infinite suspension functor with respect to under-category.
Also I noticed that the existence of the following commutative diagram, which is kind of the dual version of what I want to get:
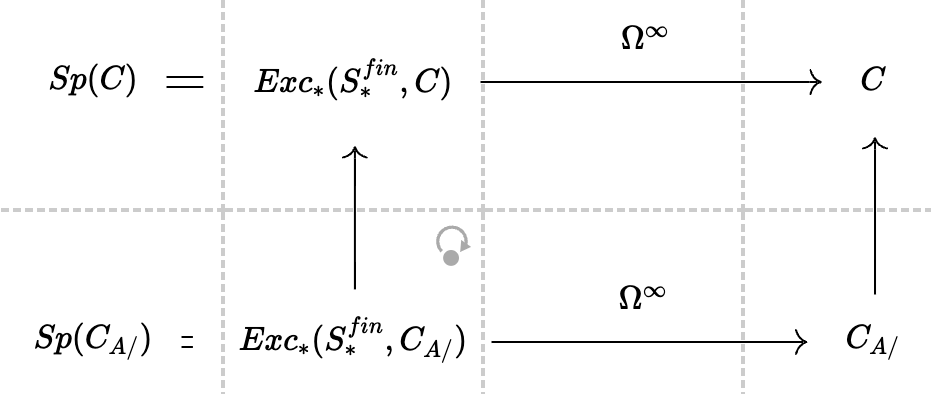
But I don't know whether there exists a mechanism to have the commutative diagram of the adjoint functor?
Any thoughts on this are very welcome!