Given a rectangle $ABCD$.
Let a Jordan curve $L_1$ joins the vertexes $A$ and $C$ and all points of $L_1$ belong the rectangle.
Let a Jordan curve $L_2$ joins the vertexes $B$ and $D$ and all points of $L_2$ belong the rectangle.
Then there exists a point of $L_1$ and $L_2$ intersection.
Has this statement a simple proof?
The statement may be connected with the Jordan curve theorem but I think its proof must be more simple.
Where one can see any proof of the statement?
Thanks.
Alternatively, wlog the rectangle $R$ is $[0,1]\times[0,1]$, and denoting $L_1$, $L_2$ the corresponding parametrization curves, one may also consider the map $$[0,1]\times[0,1]\ni (t,s)\mapsto L_1(t)-L_2(s) \in\mathbb R^2.$$ By the assumptions $L_1(0)=(0,0),L_1(1)=(1,1),L_2(0)=(0,1),L_2(1)=(0,1)$, this map is outward directed on $\partial R$, hence has a zero, by the Poincaré–Miranda theorem, which is also an easy consequence of the (planar) non-retraction theorem, or of the (planar) Brower fixed point theorem etc (and of course all of them have an easy combinatorial proof).
There's a very slick way to demonstrate this using the Hex theorem [which is known to be equivalent to the Brouwer fixed-point theorem]. In a game of Hex, two players compete to connect opposite sides of a rhombus-shaped board built out of regular hexagons. Hex cannot end in a draw: the only way for the blue player to block the red from making a connection is for blue to make a connection.
Whether we prove OP's statement for a rectangle, or for a rhombus made by adjoining two equilateral triangles, makes no difference as the two are homeomorphic. So let $A, C$ be the far corners of a rhombus with angles $60°$ and $120°$, respectively, and let $B, D$ be the near corners. The Jordan arcs $L_i$, $i = 1, 2$, are images of continuous injections $\gamma_i : [0, 1] \to ABCD$ where each $L_i$ maps $0, 1$ to opposite corners of the rhombus, $L_1$ (red) connects $A$ to $C$, $L_2$ (blue) connects $B$ to $D$.
As $L_1$ and $L_2$ are continuous images of the compact set $[0, 1]$, they are also compact subsets of the metric space $ABCD$. So to show that they intersect, it suffices to show that the distance $$d(L_1, L_2) = \inf \{ d(x, y): x \in L_1, y \in L_2 \}$$ is $0$.
This is because in any metric space, if $A$ is compact and $B$ is closed, there always exist points $x \in A$, $y \in B$ so that $d(x, y) = d(A, B)$.
But if $d(L_1, L_2) = 0$, that implies $d(x, y) = 0$ for some $x \in L_1, y \in L_2$, and it's an axiom of metric spaces that that's only possible if $x = y$, which means $x$ is also in $L_2$ and $L_1 \cap L_2$ is nonempty.
Now for the argument that $d(L_1, L_2) = 0$. For any $N \geq 2$, we may cover $ABCD$ with an $N \times N$ Hex board whose upper left corner is at $A$ and lower right corner is at $C$. For such a Hex board, the diameter of a single tile is upper bounded by $k/N$ for some absolute constant $k$. If we color all the tiles that intersect $L_1$ red, we get a red-tiled winning Hex path connecting the top to the bottom, while if we color all the tiles that intersect $L_2$ blue, we get a blue-tiled winning Hex path connecting the left to the right:
Now superimpose these two boards. If the winning path for red and the winning path for blue somehow didn't interfere with each other, that would contradict the Hex theorem. So there must be at least one purple tile that belongs to both paths:
But any purple tile must contain points from both the red Jordan arc $L_1$ and the blue Jordan arc $L_2$, which implies that $$d(L_1, L_2) \leq \text{ diameter of a single tile } \leq \frac{k}{N},$$ and since $N$ was arbitrary, that implies $d(L_1, L_2) = 0$ and the two Jordan arcs have at least one point in common. Done.
Yes, this is easier than the Jordan curve theorem. It is convenient to assume that the rectangle $ABCD$ is the unit square.
Suppose, for a contradiction, that the originally given Jordan arcs are disjoint. Using compactness of the given Jordan arcs, and a covering argument, we can replace them by nearby piecewise linear arcs. We call these $\alpha$ (connecting $A$ to $C$) and $\beta$ (connecting $B$ to $D$). Again $\alpha$ and $\beta$ are disjoint. We can also arrange for $\alpha \cup \beta$ to (a) meet the boundary of the square only at the four corners, (b) meet every vertical line in finitely many points, and (c) have at most one corner on any given vertical line. (See Pietro Majer's comment below.)
Let $(\ell_t)$ be the one-parameter family of vertical arcs cutting the square, with all points of $\ell_t$ having $x$-coordinate equal to $t$. We now partition the interval of $t$-values into finitely many pieces, depending on the combinatorics of the intersection of $\ell_t$ with $\alpha \cup \beta$. Note that $\beta$ is one side of $\alpha$ in $\ell_0$ and is on the other side of $\alpha$ in $\ell_1$. We now appeal to the combinatorial intermediate value theorem (that is, the one-dimensional version of Sperner's lemma).
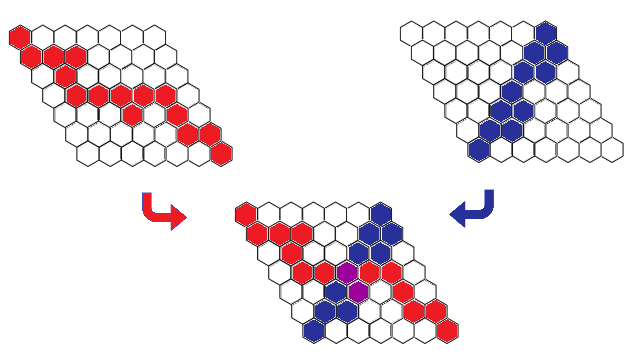
As Pietro Majer suggested in a comment, we do not have to assume that $L_1$ and $L_2$ are Jordan curves. Instead, it is enough to assume that $L_1$ is any closed connected subset of the rectangle $R:=ABCD$ containing points $A$ and $C$, while $L_2$ is any closed connected subset of $R$ containing points $B$ and $D$.
Indeed, suppose the contrary: that $L_1\cap L_2=\emptyset$. Then $L_2\subseteq R\setminus L_1$ and hence $L_2\subseteq U_2$, where $U_2$ is an open (in $R$) connected component of $R\setminus L_1$. Since $\{B,D\}\subseteq L_2\subseteq U_2$, we can find a continuous piecewise linear path $p_2\subseteq U_2$ from $B$ to $D$, and then $L_1\cap p_2=\emptyset$.
Similarly, we find a continuous piecewise linear path $p_1\subseteq R$ from $A$ to $C$ such that $p_1\cap p_2=\emptyset$. To obtain the final contradiction, we can use the reasoning in the last paragraph of Sam Nead's answer on this page. This part of the proof can also be handled by a simple, self-contained argument -- see Lemma 1 below.
It is clear now that either one of the the two connected subsets, $L_1$ and $L_2$, of $R$ can be assumed to be either closed or open in $R$.
Lemma 1: Let $l_0$ and $l_1$ be two distinct (straight) vertical lines (in $\mathbb R^2$). Let $p_1=A_0\cdots A_n$ and $p_2=B_0\cdots B_m$ be continuous piecewise linear paths (CPLPs) between $l_0$ and $l_1$ such that $\{A_0,B_0\}\subset l_0$, $\{A_n,B_m\}\subset l_1$, $A_0<B_0$ (that is, $A_0$ is strictly below $B_0$), and $B_m<A_n$. Then $p_0\cap p_1\ne\emptyset$.
Proof: Slightly moving some or all of the vertices of the CPLPs and using a compactness argument, without loss of generality (wlog) we may assume that none of the segments of the CPLPs is vertical. Morever, inserting more vertices, wlog we may assume that $m=n$ and, for each $i\in\{0,\dots,n\}$, the points $A_i$ and $B_i$ have the same abscissas, so that wlog we have either $A_i<B_i$ or $B_i<A_i$; at this key point, we used the connectedness of the CPLPs, whereby each of the CPLPs intersects every vertical line between $l_0$ and $l_1$.
Since $A_0<B_0$ and $B_n<A_n$, there is some $j\in\{0,\dots,n-1\}$ such that $A_j<B_j$ and $B_{j+1}<A_{j+1}$; for instance, we may take $j:=\max\{i\colon A_i<B_i\}$. So, the two paths intersect at a point (with abscissa between the abscissas of $A_j$ and $A_{j+1}$).
Here is a proof although I am not sure how rigorous it is. Suppose the curves don't intersect. Then imagine the rectangle as a $K_4$ graph. Since all the edges lie inside the rectangle by hypothesis, we can introduce a new vertex outside and connect it to the other four vertices of the rectangle to get a $K_5$. However this means that the resulting graph is a planar representation of $K_5$ which is impossible.
This has indeed a quick proof for Jordan arcs, if you include the usual property of separation of Jordan curves.
To avoid complications due to $L_1$ and $L_2$ possibly having other points in the boundary, we may inscribe the rectangle into (the closure of an open) disk $\Delta$, so that $\{A,B,C,D\}$ are the only points of the arcs $L_1$ and $L_2$ in the circle $\partial\Delta$.
Then the arc $L_1$ from $A$ to $C$ can be included in a closed Jordan curve, adding to it the arc $CBA$ of $\partial\Delta$. The closed Jordan curve $L_1\cup CBA$ is the boundary of some bounded connected domain $\Omega\subset\Delta$ (note that this evident inclusion needs a short explanation too, by connectedness of $\Delta$) .
The connected set $L_2\setminus \{B\}$ either meets $\partial \Omega$, necessarily in a point of $L_1$, or it is included in $\Omega$, which is not possible since $D\in L_2\setminus \{B\}$ and $\Omega\subset\Delta$, whereas $D\notin \Delta$.
