Visual proof of convergence for Steiner's symmetrization

Question

I want to find a visual proof of the following fact:

For any convex figure in the plane there is a sequence of Steiner's symmetrizations that makes it arbitrary close to a circular disc.

All proofs I know require some integral estimates. I would prefer a more visual proof (even if it is more involved).

Answer 1

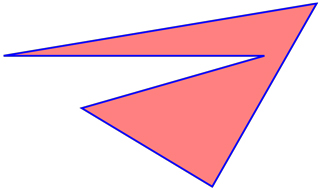
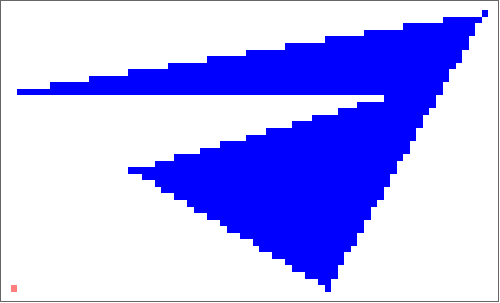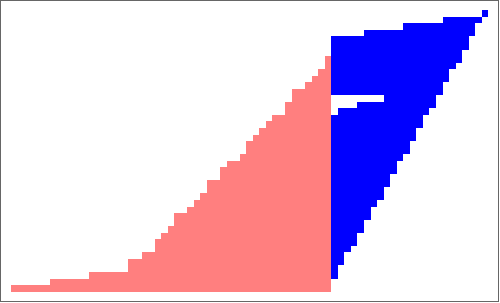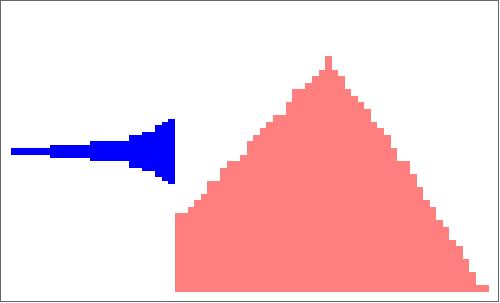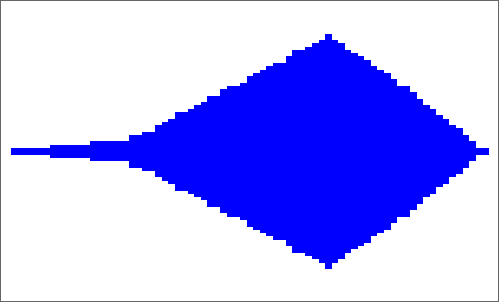
(1) Let $$ C = {\rm conv}\ B\bigcup \{x\}$$ where $B$ is a closed ball of center $o$ and $x$ is not in $B$.

Consider arc $A\subset B$, $$ \partial B \bigcap {\rm Int}\ C $$

Note that by any Steiner symmetrization $S_L$, $A$ is still in the interior ${\rm Int}\ S_L( C)$.

If $x_1$ is close to $x$ s.t. $\overrightarrow{ox}$ and $\overrightarrow{ox_1}$ are different, then $S_L(C)$ where $L=( \overrightarrow{ox_1})^\perp$ has arc $A_1$ which contains $A$. After a finite process, $\partial B-A_n$ is a one point set. Hence through a some Steiner symmetrization, $A_{n+1}=\partial B$. Hence the resulting convex and compact set contains a ball $B'$ whose radius is strictly larger than that of $B$.

(2) Fix a point $o\in \mathbb{E}^2$ which is the origin. Here assume that all hyperplane pass through $o$.

Assume that $C$ is a convex and compact subset. If $x$ is interior point in $C$, then define $v=\overrightarrow{ox}$ so that $o$ is an interior point of $S_L(C)$ for $v^\perp=L$.

In further, if $B$ is a largest closed ball in $C$ of center $o$, then there is an open arc of non-zero length in $ \partial B \bigcap {\rm Int}\ C$.

Hence we have $B'$ in $(1)$. So repeat the process.

If there is no such arc in $\partial B \bigcap {\rm Int}\ C$, then $B=C$.

Answer 2

Just for illustration, here is an animation of one step of a Steiner symmetrization applied to a rasterized version of a polygon.

---

                   

---

         
          ^{First: a histogram of vertical lengths. Second: lengths lifted to be symmetric around horizontal.}

---

Answer 3

Consider $\omega$ a convex shape and $B$ a ball having the same volume centered at a point in $\omega$ (for example the centroid). Define a coordinate system in $\Bbb{R}^d$ and make a Steiner symmetrization with respect to the $x_d$-axis which we assume contains the center of $B$.

If $\omega_S$ is the symmetrized shape then for every $t \in \Bbb{R}$: $$ |\omega_S \cap \{ x_d = t\} \cap B| \geq |\omega \cap \{x_d = t\} \cap B|.$$ The $d-1$ dimensional area contained in $B$ increases after the Steiner symmetrization for each horizontal slice orthogonal to the symmetrization direction.

Why? By definition $|\omega \cap \{x_d = t\}| = |\omega_S \cap \{ x_d = t\}|$ and $|\omega_S \cap \{x_d = t\}|$ is a $d-1$ dimensional ball. The slice at $x_d=t$ for the Steiner symmetrized shape $\omega_S$ is either fully contained in $B$ or completely covers the $d-1$ dimensional ball $B \cap \{x_d=t\}$.

Therefore the common volume $|\omega \cap B|$ increases when making a symmetrization along an axis going through the center of $B$. To make this quantitative, we need to find directions for which the common volume increases strictly:

• for example: pick a common tangent plane $\alpha$ for $B$ and $\omega$ such that $x = \alpha\cap B$ does not belong to $\omega$. Then the Steiner symmetrization with a direction normal to $\alpha$ will cover the point $x$ and will strictly increase the area $|\omega \cap B|$.